Chapter II: Electrical and magnetic properties of materials
II.1. Material
Basic structure of matter: Atoms
 Charges moving in orbits create magnetic fields.
Charges moving in orbits create magnetic fields.
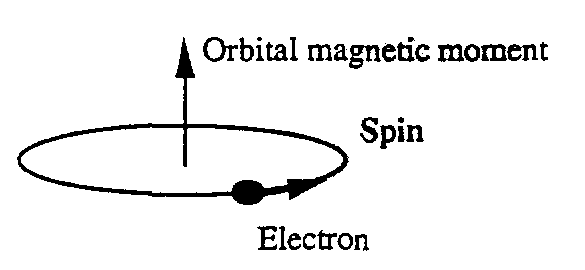 And these electrons move in orbits around the nucleus creating a magnetic field. That rotation of the electron is the magnetic moment produced by the electron revolving around itself. The nuclei are located in a lattice structure. The form of the lattice depends on the balance between different forces: electric - magnetic - kinetic energy (oscillation-rotation) - attraction of matter. That explains about different lattices and affects the crystal structure of different atoms when we make alloys. As for the electrical and magnetic properties of matter, it is evident that the interaction between matter and the external magnetic field will depend on the electro-magnetic balance of the crystal structure. In the table below are the crystal structures of some metals.
And these electrons move in orbits around the nucleus creating a magnetic field. That rotation of the electron is the magnetic moment produced by the electron revolving around itself. The nuclei are located in a lattice structure. The form of the lattice depends on the balance between different forces: electric - magnetic - kinetic energy (oscillation-rotation) - attraction of matter. That explains about different lattices and affects the crystal structure of different atoms when we make alloys. As for the electrical and magnetic properties of matter, it is evident that the interaction between matter and the external magnetic field will depend on the electro-magnetic balance of the crystal structure. In the table below are the crystal structures of some metals.
 When alloys of substances are made, we have a different lattice structure, a new electromagnetic equilibrium. In addition, when alloys of substances are made, we cannot be sure of their interaction with electric or magnetic fields.
II.2. Duality of ELECTROLOGY
We talk about electromagnetism because the generated electric field affects the magnetic field and vice versa. For example: Charge when moving will create a magnetic field perpendicular to the orbit. When the changing magnetic field approaches the conductor, the induced current will appear perpendicular to the lines of force of the magnetic field. Electricity and Magnetic Field are always perpendicular to each other.
II.3. Electrical properties of materials
Metallic materials are defined by their ability to allow movement of positively or negatively charged particles.
When alloys of substances are made, we have a different lattice structure, a new electromagnetic equilibrium. In addition, when alloys of substances are made, we cannot be sure of their interaction with electric or magnetic fields.
II.2. Duality of ELECTROLOGY
We talk about electromagnetism because the generated electric field affects the magnetic field and vice versa. For example: Charge when moving will create a magnetic field perpendicular to the orbit. When the changing magnetic field approaches the conductor, the induced current will appear perpendicular to the lines of force of the magnetic field. Electricity and Magnetic Field are always perpendicular to each other.
II.3. Electrical properties of materials
Metallic materials are defined by their ability to allow movement of positively or negatively charged particles.
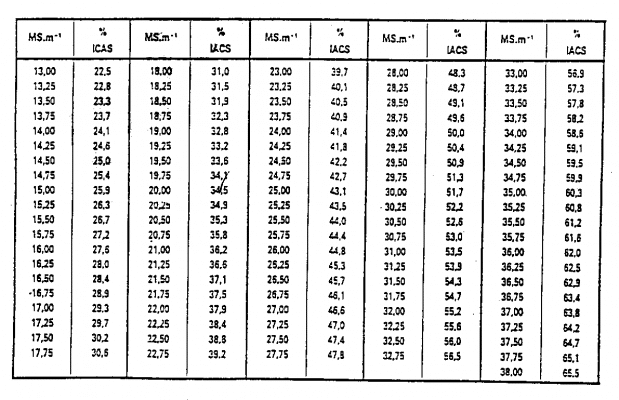
 Electrical properties of materials are defined from two quantities called conductivity, expressed in Siemens/meter (S/m) Conductivity is achieved by the movement of free electrons in a conductor. . In practice, Conductivity expressed in Mega-Siemens/meter (MS/m) 1 MS/m = 1,000,000 S/m • is called resistivity, expressed in Ohm-meters (. m). We have a relationship: = 1/ The electrical conductivity of a material depends on its nature and temperature. It also depends on the heat or mechanical treatment of the material. For example: • Conductivity of pure copper: = 58 MS/m • Conductivity of aluminum alloy 15 MS/m 35 MS/m • Conductivity of titanium alloy: 2 MS/m • Conductivity of iron: 1 MS/m 9 MS/m The change in conductivity with temperature is expressed by the formula formula: = o / (1 + (T – To)) Conductivity at temperature To Conductivity at temperature T a coefficient that depends on the nature of the material Example coefficient of aluminum alloy about 0.003 to 0.004 when the temperature is in the range 0 C to 100 C Note: The electrical conductivity of the material can be expressed as % IACS (International Annealed Copper Standard) where pure copper is taken as the witness. The conductivity of the material is then expressed as % the conductivity of pure copper. In that unit, the conductivity of pure copper: = 58 MS/m = 100 % IACS. We have: 1 % IACS = 0.58 MS/m 1 MS/m = 1.7241 % IACS The following table provides the corresponding values between conductivity expressed in international system units and % IACS units.
Electrical properties of materials are defined from two quantities called conductivity, expressed in Siemens/meter (S/m) Conductivity is achieved by the movement of free electrons in a conductor. . In practice, Conductivity expressed in Mega-Siemens/meter (MS/m) 1 MS/m = 1,000,000 S/m • is called resistivity, expressed in Ohm-meters (. m). We have a relationship: = 1/ The electrical conductivity of a material depends on its nature and temperature. It also depends on the heat or mechanical treatment of the material. For example: • Conductivity of pure copper: = 58 MS/m • Conductivity of aluminum alloy 15 MS/m 35 MS/m • Conductivity of titanium alloy: 2 MS/m • Conductivity of iron: 1 MS/m 9 MS/m The change in conductivity with temperature is expressed by the formula formula: = o / (1 + (T – To)) Conductivity at temperature To Conductivity at temperature T a coefficient that depends on the nature of the material Example coefficient of aluminum alloy about 0.003 to 0.004 when the temperature is in the range 0 C to 100 C Note: The electrical conductivity of the material can be expressed as % IACS (International Annealed Copper Standard) where pure copper is taken as the witness. The conductivity of the material is then expressed as % the conductivity of pure copper. In that unit, the conductivity of pure copper: = 58 MS/m = 100 % IACS. We have: 1 % IACS = 0.58 MS/m 1 MS/m = 1.7241 % IACS The following table provides the corresponding values between conductivity expressed in international system units and % IACS units.
 II.4. Magnetic properties of materials
The magnetic properties of a material are determined by the influence of the H magnetic field present in the material. To quantify this effect, we rely on two basic concepts: • Permeability • Magnetism
4.1. Magnitude of permeability
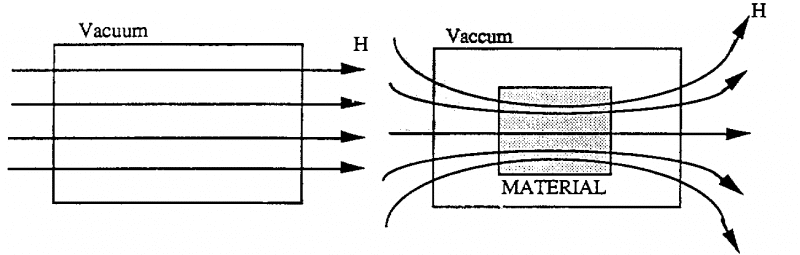
Magnetic permeability is understood as a quantity that characterizes the ability of a material to 'carry, conduct' the lines of force of the magnetic field. It is related to the growth ability of magnetized materials. Magnetic permeability has the unit of measurement is Henry/meter (H/m).
II.4. Magnetic properties of materials
The magnetic properties of a material are determined by the influence of the H magnetic field present in the material. To quantify this effect, we rely on two basic concepts: • Permeability • Magnetism
4.1. Magnitude of permeability
Magnetic permeability is understood as a quantity that characterizes the ability of a material to 'carry, conduct' the lines of force of the magnetic field. It is related to the growth ability of magnetized materials. Magnetic permeability has the unit of measurement is Henry/meter (H/m).
 4.2. Magnetic Inductance
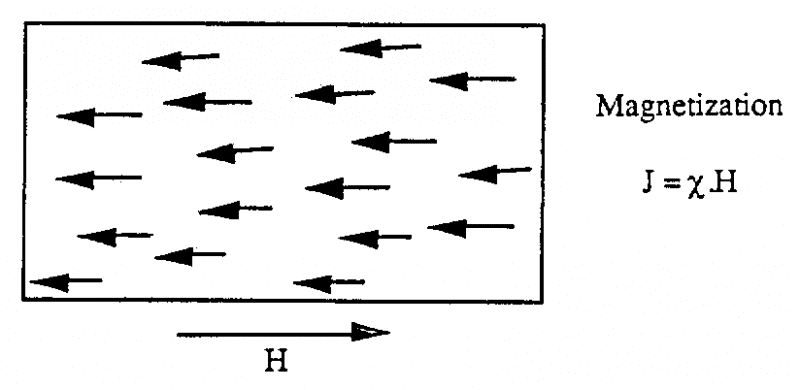
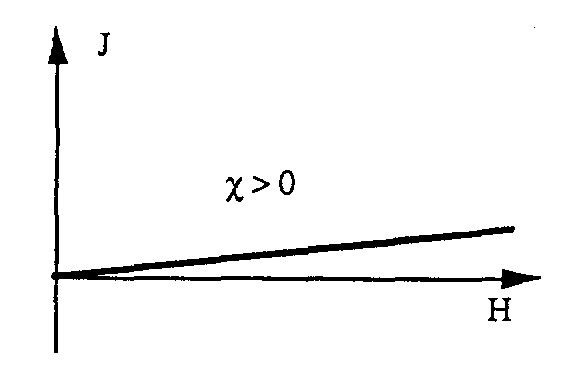
When the material is subjected to the H magnetic field, it is found that the material can produce a J Magnetization. The relationship between the J Magnetization and the H magnetic field can be expressed by the following formula: J = . H Where is called the inductance, a dimensionless (unitless) quantity H and J are measured in Amperes/meter (A/m) This relationship shows that the material is susceptible to magnetic fields , the ability to react to the impact of a magnetic field.
4.3. Inductive
We call magnetic induction B the quantity representing the magnetic flux (Magnetic flux) through the material. The unit of magnetic induction B is Tesla (T). In a vacuum, the magnetic induction B and the magnetic field H are related by: B = 0. H Where 0 is the vacuum permeability, 0 = 4..10-7 H/m When the H magnetic field passes through the material, the magnetic induction B is calculated as follows: B = 0. (H+J) B = 0. (H+ .H) B = 0. (1+). H Set r = (1+) We have: B = 0. r . H = . H In which . is the permeability of the material r is the relative permeability (dimensionless) r = /0 Generally we classify materials into 3 categories according to the way they react to the magnetic field: • Materials Paramagnetic • Paramagnetic materials • Ferromagnetic materials Note: Paramagnetic and paramagnetic materials are also called non-magnetic materials because they have very weak magnetic susceptibility compared to ferromagnetic materials o Paramagnetic materials and paramagnetic materials have very weak coercivity ( 1) r = 1 o Ferromagnetic materials have high coercivity: r 1
4.4. Antimagnetic materials
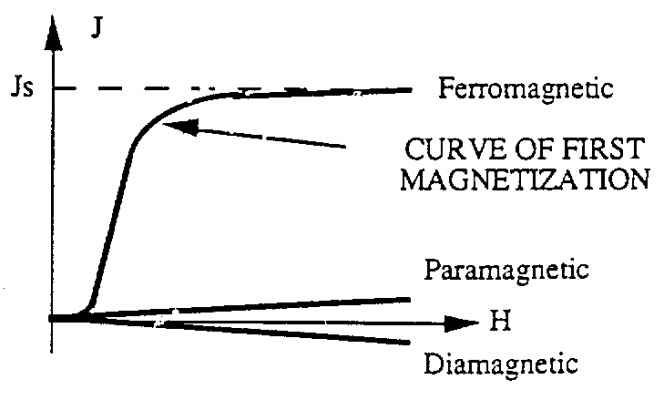
For diamagnetic materials, in the absence of a magnetic field, the orbits of the electrons around the nucleus are arbitrary and the sum of the magnetic field orbits produced by the electron's motion is zero (cancellation). – null). We say that the material has 2 cancel (zero) atomic magnetic moments. When these materials are subjected to the H magnetic field, the electron orbits tend to be in a direction perpendicular to the direction of the H magnetic field. In this case the sum of the orbital magnetic moments created by the electron movement non-zero (non-destructive). For each atom, we say that they have a magnetic moment. The sum of the magnetic moments of the atoms imparting a magnetization J into the material.
4.2. Magnetic Inductance
When the material is subjected to the H magnetic field, it is found that the material can produce a J Magnetization. The relationship between the J Magnetization and the H magnetic field can be expressed by the following formula: J = . H Where is called the inductance, a dimensionless (unitless) quantity H and J are measured in Amperes/meter (A/m) This relationship shows that the material is susceptible to magnetic fields , the ability to react to the impact of a magnetic field.
4.3. Inductive
We call magnetic induction B the quantity representing the magnetic flux (Magnetic flux) through the material. The unit of magnetic induction B is Tesla (T). In a vacuum, the magnetic induction B and the magnetic field H are related by: B = 0. H Where 0 is the vacuum permeability, 0 = 4..10-7 H/m When the H magnetic field passes through the material, the magnetic induction B is calculated as follows: B = 0. (H+J) B = 0. (H+ .H) B = 0. (1+). H Set r = (1+) We have: B = 0. r . H = . H In which . is the permeability of the material r is the relative permeability (dimensionless) r = /0 Generally we classify materials into 3 categories according to the way they react to the magnetic field: • Materials Paramagnetic • Paramagnetic materials • Ferromagnetic materials Note: Paramagnetic and paramagnetic materials are also called non-magnetic materials because they have very weak magnetic susceptibility compared to ferromagnetic materials o Paramagnetic materials and paramagnetic materials have very weak coercivity ( 1) r = 1 o Ferromagnetic materials have high coercivity: r 1
4.4. Antimagnetic materials
For diamagnetic materials, in the absence of a magnetic field, the orbits of the electrons around the nucleus are arbitrary and the sum of the magnetic field orbits produced by the electron's motion is zero (cancellation). – null). We say that the material has 2 cancel (zero) atomic magnetic moments. When these materials are subjected to the H magnetic field, the electron orbits tend to be in a direction perpendicular to the direction of the H magnetic field. In this case the sum of the orbital magnetic moments created by the electron movement non-zero (non-destructive). For each atom, we say that they have a magnetic moment. The sum of the magnetic moments of the atoms imparting a magnetization J into the material.
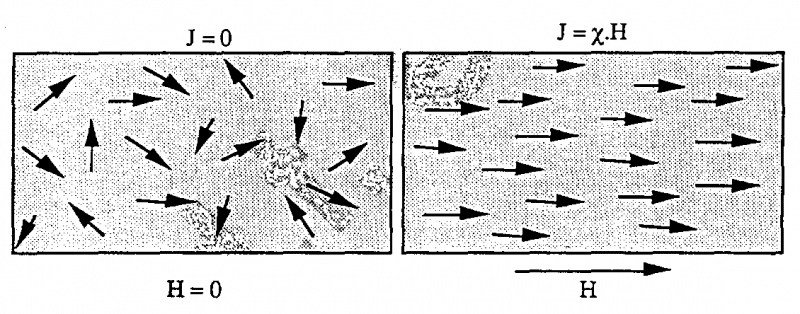
 The direction of the sum of the magnetic moments of the atoms is always opposite to the direction of the magnetic field H. The diamagnetic material is characterized by its weak inductance and has a negative sign ( 0).
The direction of the sum of the magnetic moments of the atoms is always opposite to the direction of the magnetic field H. The diamagnetic material is characterized by its weak inductance and has a negative sign ( 0).
 Examples of some diamagnetic materials: Lead Silver Mercury Copper
4.5. Paramagnetic materials
For paramagnetic materials, the atomic magnetic moments are non-zero but in the absence of the H magnetic field they have different directions and the total moment is zero.
Examples of some diamagnetic materials: Lead Silver Mercury Copper
4.5. Paramagnetic materials
For paramagnetic materials, the atomic magnetic moments are non-zero but in the absence of the H magnetic field they have different directions and the total moment is zero.
 Under the influence of the magnetic field H, the moment tends to follow the magnetic field H, resulting in magnetization J. Paramagnetic materials are characterized by weak coercivity and have a positive sign ( 0).
Under the influence of the magnetic field H, the moment tends to follow the magnetic field H, resulting in magnetization J. Paramagnetic materials are characterized by weak coercivity and have a positive sign ( 0).
 Examples of some paramagnetic materials Platinum Aluminum Chromium Manganese
Examples of some paramagnetic materials Platinum Aluminum Chromium Manganese
 4.6. Ferromagnetic materials
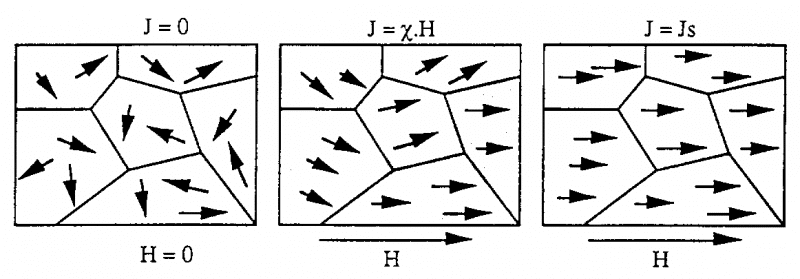
Ferromagnetic materials are characterized by the appearance of magnetic domains (domains) These domains have a very small area in the material (side size is about 10 m), their magnetic moment self-generates the same magnetization. direction when the material is placed in a magnetic field H For ferromagnetic materials that have not been magnetized (magnetic), the result of the magnetization of the different magnetic regions is zero.
4.6. Ferromagnetic materials
Ferromagnetic materials are characterized by the appearance of magnetic domains (domains) These domains have a very small area in the material (side size is about 10 m), their magnetic moment self-generates the same magnetization. direction when the material is placed in a magnetic field H For ferromagnetic materials that have not been magnetized (magnetic), the result of the magnetization of the different magnetic regions is zero.
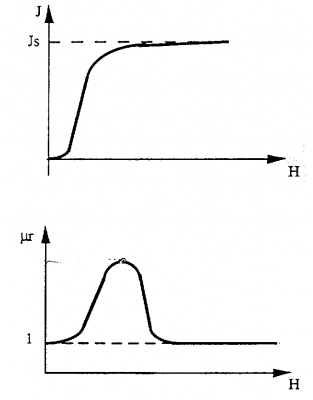
 When these materials are subjected to a magnetic field, they are able to develop strong magnetization. The occurrence of such magnetization is related to the change in the size of the magnetic regions as well as the direction of the magnetic moments with the magnetic field H For these materials, the value of magnetization J does not vary linearly with the magnetic field H. The obtained curve is called the first magnetization curve.
When these materials are subjected to a magnetic field, they are able to develop strong magnetization. The occurrence of such magnetization is related to the change in the size of the magnetic regions as well as the direction of the magnetic moments with the magnetic field H For these materials, the value of magnetization J does not vary linearly with the magnetic field H. The obtained curve is called the first magnetization curve.
 When all magnetic moments are in the direction of the magnetic field H, we say they have saturation magnetization. Examples of some ferromagnetic materials • Nickel • Cobalt • Iron • Steel
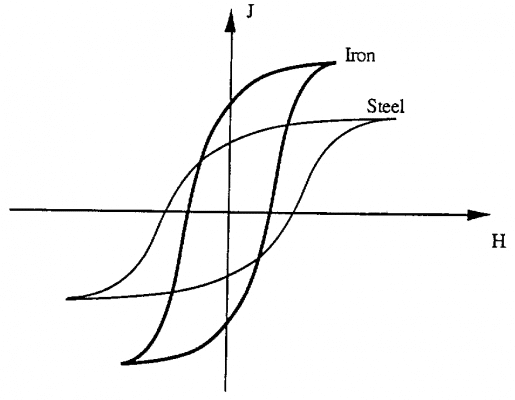
II.5. Remnant and hysteresis loops
We call residual magnetism the amount of magnetic residue remaining in a material after being placed in a magnetic field H. Paramagnetic and paramagnetic materials are characterized by the fact that they have no residual magnetism. The induced magnetization in the material disappears as soon as we remove the H magnetic field. This phenomenon does not occur in the case of ferromagnetic materials. When the magnetic field decreases from +Hm value, saturates Js to value 0. We draw a curve from saturation value to value from residual Jr (segment AJr)
When all magnetic moments are in the direction of the magnetic field H, we say they have saturation magnetization. Examples of some ferromagnetic materials • Nickel • Cobalt • Iron • Steel
II.5. Remnant and hysteresis loops
We call residual magnetism the amount of magnetic residue remaining in a material after being placed in a magnetic field H. Paramagnetic and paramagnetic materials are characterized by the fact that they have no residual magnetism. The induced magnetization in the material disappears as soon as we remove the H magnetic field. This phenomenon does not occur in the case of ferromagnetic materials. When the magnetic field decreases from +Hm value, saturates Js to value 0. We draw a curve from saturation value to value from residual Jr (segment AJr)
 Jr+ is called the residual magnetism In case we want to limit the amount of that Jr residual, the magnetic field can be applied in the opposite direction (H 0) with the magnetic field applied at the first magnetization. Then we draw segment AB. The residual amount is removed (J = 0) when the magnetic field H reaches the value Hc.
Jr+ is called the residual magnetism In case we want to limit the amount of that Jr residual, the magnetic field can be applied in the opposite direction (H 0) with the magnetic field applied at the first magnetization. Then we draw segment AB. The residual amount is removed (J = 0) when the magnetic field H reaches the value Hc.
 That value is called the forced magnetic field. If we keep changing from -Hc value to -Hm value, we again reach new saturation value –Js (BC segment)
That value is called the forced magnetic field. If we keep changing from -Hc value to -Hm value, we again reach new saturation value –Js (BC segment)
 If we now increase the magnetic field from -Hm to the value Hm, we plot the CDA curve.
If we now increase the magnetic field from -Hm to the value Hm, we plot the CDA curve.
 The closed curve ABCD, obtained with the change of the magnetic field H between the values of Hm and –Hm is called the hysteresis loop. The shape of the hysteresis ring depends on many factors, including the nature and composition of the ferromagnetic material and the mechanical processing; heat treatment of materials. Therefore, there are ferromagnetic types with large residual magnetization, while other types have small residual magnetization.
The closed curve ABCD, obtained with the change of the magnetic field H between the values of Hm and –Hm is called the hysteresis loop. The shape of the hysteresis ring depends on many factors, including the nature and composition of the ferromagnetic material and the mechanical processing; heat treatment of materials. Therefore, there are ferromagnetic types with large residual magnetization, while other types have small residual magnetization.
 II.6. Curie temperature
Any ferromagnetic material containing residual magnetism can be demagnetized by heating it to a suitable temperature and then cooling it in the absence of a magnetic field. The temperature at which a material changes its properties from a magnetic state to a non-magnetic (paramagnetic) state is called the temperature or Curie point. That temperature depends on the properties and composition of the material. For example, a Nickel alloy containing 1 % Silicon has a Curie temperature of 3200C. Nickel alloy containing 5% Silicon will have a Curie temperature of 450C. Curie temperature of steel varies from 6500C to 8700C. This phenomenon is reversible. When the temperature of the material is higher than the Curie Temperature, the material becomes paramagnetic. When the temperature is lower than the Curie Temperature, the material becomes ferromagnetic again but loses all its residual magnetism.
II.6. Curie temperature
Any ferromagnetic material containing residual magnetism can be demagnetized by heating it to a suitable temperature and then cooling it in the absence of a magnetic field. The temperature at which a material changes its properties from a magnetic state to a non-magnetic (paramagnetic) state is called the temperature or Curie point. That temperature depends on the properties and composition of the material. For example, a Nickel alloy containing 1 % Silicon has a Curie temperature of 3200C. Nickel alloy containing 5% Silicon will have a Curie temperature of 450C. Curie temperature of steel varies from 6500C to 8700C. This phenomenon is reversible. When the temperature of the material is higher than the Curie Temperature, the material becomes paramagnetic. When the temperature is lower than the Curie Temperature, the material becomes ferromagnetic again but loses all its residual magnetism.
 Charges moving in orbits create magnetic fields.
Charges moving in orbits create magnetic fields.
 And these electrons move in orbits around the nucleus creating a magnetic field. That rotation of the electron is the magnetic moment produced by the electron revolving around itself. The nuclei are located in a lattice structure. The form of the lattice depends on the balance between different forces: electric - magnetic - kinetic energy (oscillation-rotation) - attraction of matter. That explains about different lattices and affects the crystal structure of different atoms when we make alloys. As for the electrical and magnetic properties of matter, it is evident that the interaction between matter and the external magnetic field will depend on the electro-magnetic balance of the crystal structure. In the table below are the crystal structures of some metals.
And these electrons move in orbits around the nucleus creating a magnetic field. That rotation of the electron is the magnetic moment produced by the electron revolving around itself. The nuclei are located in a lattice structure. The form of the lattice depends on the balance between different forces: electric - magnetic - kinetic energy (oscillation-rotation) - attraction of matter. That explains about different lattices and affects the crystal structure of different atoms when we make alloys. As for the electrical and magnetic properties of matter, it is evident that the interaction between matter and the external magnetic field will depend on the electro-magnetic balance of the crystal structure. In the table below are the crystal structures of some metals.
 When alloys of substances are made, we have a different lattice structure, a new electromagnetic equilibrium. In addition, when alloys of substances are made, we cannot be sure of their interaction with electric or magnetic fields.
II.2. Duality of ELECTROLOGY
We talk about electromagnetism because the generated electric field affects the magnetic field and vice versa. For example: Charge when moving will create a magnetic field perpendicular to the orbit. When the changing magnetic field approaches the conductor, the induced current will appear perpendicular to the lines of force of the magnetic field. Electricity and Magnetic Field are always perpendicular to each other.
II.3. Electrical properties of materials
Metallic materials are defined by their ability to allow movement of positively or negatively charged particles.
When alloys of substances are made, we have a different lattice structure, a new electromagnetic equilibrium. In addition, when alloys of substances are made, we cannot be sure of their interaction with electric or magnetic fields.
II.2. Duality of ELECTROLOGY
We talk about electromagnetism because the generated electric field affects the magnetic field and vice versa. For example: Charge when moving will create a magnetic field perpendicular to the orbit. When the changing magnetic field approaches the conductor, the induced current will appear perpendicular to the lines of force of the magnetic field. Electricity and Magnetic Field are always perpendicular to each other.
II.3. Electrical properties of materials
Metallic materials are defined by their ability to allow movement of positively or negatively charged particles.
 Electrical properties of materials are defined from two quantities called conductivity, expressed in Siemens/meter (S/m) Conductivity is achieved by the movement of free electrons in a conductor. . In practice, Conductivity expressed in Mega-Siemens/meter (MS/m) 1 MS/m = 1,000,000 S/m • is called resistivity, expressed in Ohm-meters (. m). We have a relationship: = 1/ The electrical conductivity of a material depends on its nature and temperature. It also depends on the heat or mechanical treatment of the material. For example: • Conductivity of pure copper: = 58 MS/m • Conductivity of aluminum alloy 15 MS/m 35 MS/m • Conductivity of titanium alloy: 2 MS/m • Conductivity of iron: 1 MS/m 9 MS/m The change in conductivity with temperature is expressed by the formula formula: = o / (1 + (T – To)) Conductivity at temperature To Conductivity at temperature T a coefficient that depends on the nature of the material Example coefficient of aluminum alloy about 0.003 to 0.004 when the temperature is in the range 0 C to 100 C Note: The electrical conductivity of the material can be expressed as % IACS (International Annealed Copper Standard) where pure copper is taken as the witness. The conductivity of the material is then expressed as % the conductivity of pure copper. In that unit, the conductivity of pure copper: = 58 MS/m = 100 % IACS. We have: 1 % IACS = 0.58 MS/m 1 MS/m = 1.7241 % IACS The following table provides the corresponding values between conductivity expressed in international system units and % IACS units.
Electrical properties of materials are defined from two quantities called conductivity, expressed in Siemens/meter (S/m) Conductivity is achieved by the movement of free electrons in a conductor. . In practice, Conductivity expressed in Mega-Siemens/meter (MS/m) 1 MS/m = 1,000,000 S/m • is called resistivity, expressed in Ohm-meters (. m). We have a relationship: = 1/ The electrical conductivity of a material depends on its nature and temperature. It also depends on the heat or mechanical treatment of the material. For example: • Conductivity of pure copper: = 58 MS/m • Conductivity of aluminum alloy 15 MS/m 35 MS/m • Conductivity of titanium alloy: 2 MS/m • Conductivity of iron: 1 MS/m 9 MS/m The change in conductivity with temperature is expressed by the formula formula: = o / (1 + (T – To)) Conductivity at temperature To Conductivity at temperature T a coefficient that depends on the nature of the material Example coefficient of aluminum alloy about 0.003 to 0.004 when the temperature is in the range 0 C to 100 C Note: The electrical conductivity of the material can be expressed as % IACS (International Annealed Copper Standard) where pure copper is taken as the witness. The conductivity of the material is then expressed as % the conductivity of pure copper. In that unit, the conductivity of pure copper: = 58 MS/m = 100 % IACS. We have: 1 % IACS = 0.58 MS/m 1 MS/m = 1.7241 % IACS The following table provides the corresponding values between conductivity expressed in international system units and % IACS units.
 II.4. Magnetic properties of materials
The magnetic properties of a material are determined by the influence of the H magnetic field present in the material. To quantify this effect, we rely on two basic concepts: • Permeability • Magnetism
4.1. Magnitude of permeability
Magnetic permeability is understood as a quantity that characterizes the ability of a material to 'carry, conduct' the lines of force of the magnetic field. It is related to the growth ability of magnetized materials. Magnetic permeability has the unit of measurement is Henry/meter (H/m).
II.4. Magnetic properties of materials
The magnetic properties of a material are determined by the influence of the H magnetic field present in the material. To quantify this effect, we rely on two basic concepts: • Permeability • Magnetism
4.1. Magnitude of permeability
Magnetic permeability is understood as a quantity that characterizes the ability of a material to 'carry, conduct' the lines of force of the magnetic field. It is related to the growth ability of magnetized materials. Magnetic permeability has the unit of measurement is Henry/meter (H/m).
 4.2. Magnetic Inductance
When the material is subjected to the H magnetic field, it is found that the material can produce a J Magnetization. The relationship between the J Magnetization and the H magnetic field can be expressed by the following formula: J = . H Where is called the inductance, a dimensionless (unitless) quantity H and J are measured in Amperes/meter (A/m) This relationship shows that the material is susceptible to magnetic fields , the ability to react to the impact of a magnetic field.
4.3. Inductive
We call magnetic induction B the quantity representing the magnetic flux (Magnetic flux) through the material. The unit of magnetic induction B is Tesla (T). In a vacuum, the magnetic induction B and the magnetic field H are related by: B = 0. H Where 0 is the vacuum permeability, 0 = 4..10-7 H/m When the H magnetic field passes through the material, the magnetic induction B is calculated as follows: B = 0. (H+J) B = 0. (H+ .H) B = 0. (1+). H Set r = (1+) We have: B = 0. r . H = . H In which . is the permeability of the material r is the relative permeability (dimensionless) r = /0 Generally we classify materials into 3 categories according to the way they react to the magnetic field: • Materials Paramagnetic • Paramagnetic materials • Ferromagnetic materials Note: Paramagnetic and paramagnetic materials are also called non-magnetic materials because they have very weak magnetic susceptibility compared to ferromagnetic materials o Paramagnetic materials and paramagnetic materials have very weak coercivity ( 1) r = 1 o Ferromagnetic materials have high coercivity: r 1
4.4. Antimagnetic materials
For diamagnetic materials, in the absence of a magnetic field, the orbits of the electrons around the nucleus are arbitrary and the sum of the magnetic field orbits produced by the electron's motion is zero (cancellation). – null). We say that the material has 2 cancel (zero) atomic magnetic moments. When these materials are subjected to the H magnetic field, the electron orbits tend to be in a direction perpendicular to the direction of the H magnetic field. In this case the sum of the orbital magnetic moments created by the electron movement non-zero (non-destructive). For each atom, we say that they have a magnetic moment. The sum of the magnetic moments of the atoms imparting a magnetization J into the material.
4.2. Magnetic Inductance
When the material is subjected to the H magnetic field, it is found that the material can produce a J Magnetization. The relationship between the J Magnetization and the H magnetic field can be expressed by the following formula: J = . H Where is called the inductance, a dimensionless (unitless) quantity H and J are measured in Amperes/meter (A/m) This relationship shows that the material is susceptible to magnetic fields , the ability to react to the impact of a magnetic field.
4.3. Inductive
We call magnetic induction B the quantity representing the magnetic flux (Magnetic flux) through the material. The unit of magnetic induction B is Tesla (T). In a vacuum, the magnetic induction B and the magnetic field H are related by: B = 0. H Where 0 is the vacuum permeability, 0 = 4..10-7 H/m When the H magnetic field passes through the material, the magnetic induction B is calculated as follows: B = 0. (H+J) B = 0. (H+ .H) B = 0. (1+). H Set r = (1+) We have: B = 0. r . H = . H In which . is the permeability of the material r is the relative permeability (dimensionless) r = /0 Generally we classify materials into 3 categories according to the way they react to the magnetic field: • Materials Paramagnetic • Paramagnetic materials • Ferromagnetic materials Note: Paramagnetic and paramagnetic materials are also called non-magnetic materials because they have very weak magnetic susceptibility compared to ferromagnetic materials o Paramagnetic materials and paramagnetic materials have very weak coercivity ( 1) r = 1 o Ferromagnetic materials have high coercivity: r 1
4.4. Antimagnetic materials
For diamagnetic materials, in the absence of a magnetic field, the orbits of the electrons around the nucleus are arbitrary and the sum of the magnetic field orbits produced by the electron's motion is zero (cancellation). – null). We say that the material has 2 cancel (zero) atomic magnetic moments. When these materials are subjected to the H magnetic field, the electron orbits tend to be in a direction perpendicular to the direction of the H magnetic field. In this case the sum of the orbital magnetic moments created by the electron movement non-zero (non-destructive). For each atom, we say that they have a magnetic moment. The sum of the magnetic moments of the atoms imparting a magnetization J into the material.
 The direction of the sum of the magnetic moments of the atoms is always opposite to the direction of the magnetic field H. The diamagnetic material is characterized by its weak inductance and has a negative sign ( 0).
The direction of the sum of the magnetic moments of the atoms is always opposite to the direction of the magnetic field H. The diamagnetic material is characterized by its weak inductance and has a negative sign ( 0).
 Examples of some diamagnetic materials: Lead Silver Mercury Copper
4.5. Paramagnetic materials
For paramagnetic materials, the atomic magnetic moments are non-zero but in the absence of the H magnetic field they have different directions and the total moment is zero.
Examples of some diamagnetic materials: Lead Silver Mercury Copper
4.5. Paramagnetic materials
For paramagnetic materials, the atomic magnetic moments are non-zero but in the absence of the H magnetic field they have different directions and the total moment is zero.
 Under the influence of the magnetic field H, the moment tends to follow the magnetic field H, resulting in magnetization J. Paramagnetic materials are characterized by weak coercivity and have a positive sign ( 0).
Under the influence of the magnetic field H, the moment tends to follow the magnetic field H, resulting in magnetization J. Paramagnetic materials are characterized by weak coercivity and have a positive sign ( 0).
 Examples of some paramagnetic materials Platinum Aluminum Chromium Manganese
Examples of some paramagnetic materials Platinum Aluminum Chromium Manganese
 4.6. Ferromagnetic materials
Ferromagnetic materials are characterized by the appearance of magnetic domains (domains) These domains have a very small area in the material (side size is about 10 m), their magnetic moment self-generates the same magnetization. direction when the material is placed in a magnetic field H For ferromagnetic materials that have not been magnetized (magnetic), the result of the magnetization of the different magnetic regions is zero.
4.6. Ferromagnetic materials
Ferromagnetic materials are characterized by the appearance of magnetic domains (domains) These domains have a very small area in the material (side size is about 10 m), their magnetic moment self-generates the same magnetization. direction when the material is placed in a magnetic field H For ferromagnetic materials that have not been magnetized (magnetic), the result of the magnetization of the different magnetic regions is zero.
 When these materials are subjected to a magnetic field, they are able to develop strong magnetization. The occurrence of such magnetization is related to the change in the size of the magnetic regions as well as the direction of the magnetic moments with the magnetic field H For these materials, the value of magnetization J does not vary linearly with the magnetic field H. The obtained curve is called the first magnetization curve.
When these materials are subjected to a magnetic field, they are able to develop strong magnetization. The occurrence of such magnetization is related to the change in the size of the magnetic regions as well as the direction of the magnetic moments with the magnetic field H For these materials, the value of magnetization J does not vary linearly with the magnetic field H. The obtained curve is called the first magnetization curve.
 When all magnetic moments are in the direction of the magnetic field H, we say they have saturation magnetization. Examples of some ferromagnetic materials • Nickel • Cobalt • Iron • Steel
II.5. Remnant and hysteresis loops
We call residual magnetism the amount of magnetic residue remaining in a material after being placed in a magnetic field H. Paramagnetic and paramagnetic materials are characterized by the fact that they have no residual magnetism. The induced magnetization in the material disappears as soon as we remove the H magnetic field. This phenomenon does not occur in the case of ferromagnetic materials. When the magnetic field decreases from +Hm value, saturates Js to value 0. We draw a curve from saturation value to value from residual Jr (segment AJr)
When all magnetic moments are in the direction of the magnetic field H, we say they have saturation magnetization. Examples of some ferromagnetic materials • Nickel • Cobalt • Iron • Steel
II.5. Remnant and hysteresis loops
We call residual magnetism the amount of magnetic residue remaining in a material after being placed in a magnetic field H. Paramagnetic and paramagnetic materials are characterized by the fact that they have no residual magnetism. The induced magnetization in the material disappears as soon as we remove the H magnetic field. This phenomenon does not occur in the case of ferromagnetic materials. When the magnetic field decreases from +Hm value, saturates Js to value 0. We draw a curve from saturation value to value from residual Jr (segment AJr)
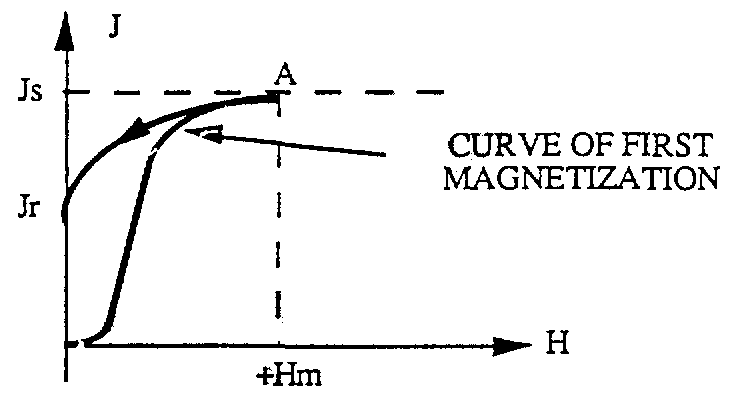 Jr+ is called the residual magnetism In case we want to limit the amount of that Jr residual, the magnetic field can be applied in the opposite direction (H 0) with the magnetic field applied at the first magnetization. Then we draw segment AB. The residual amount is removed (J = 0) when the magnetic field H reaches the value Hc.
Jr+ is called the residual magnetism In case we want to limit the amount of that Jr residual, the magnetic field can be applied in the opposite direction (H 0) with the magnetic field applied at the first magnetization. Then we draw segment AB. The residual amount is removed (J = 0) when the magnetic field H reaches the value Hc.
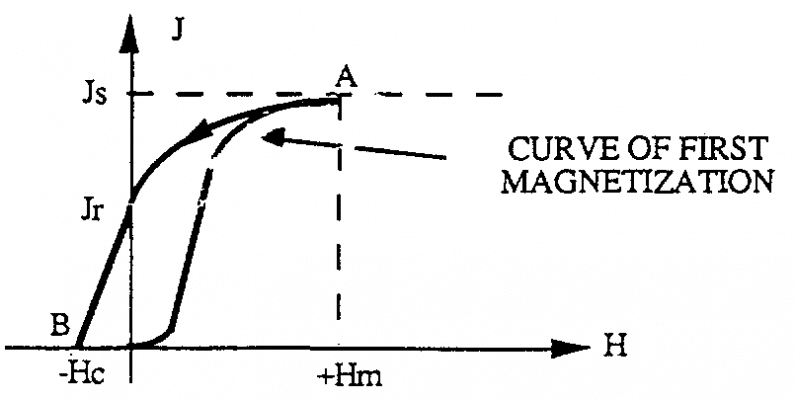 That value is called the forced magnetic field. If we keep changing from -Hc value to -Hm value, we again reach new saturation value –Js (BC segment)
That value is called the forced magnetic field. If we keep changing from -Hc value to -Hm value, we again reach new saturation value –Js (BC segment)
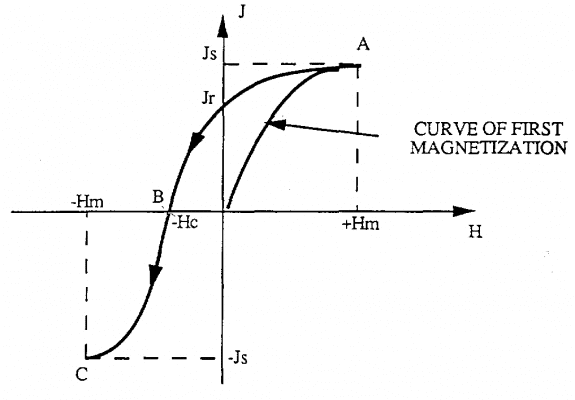 If we now increase the magnetic field from -Hm to the value Hm, we plot the CDA curve.
If we now increase the magnetic field from -Hm to the value Hm, we plot the CDA curve.
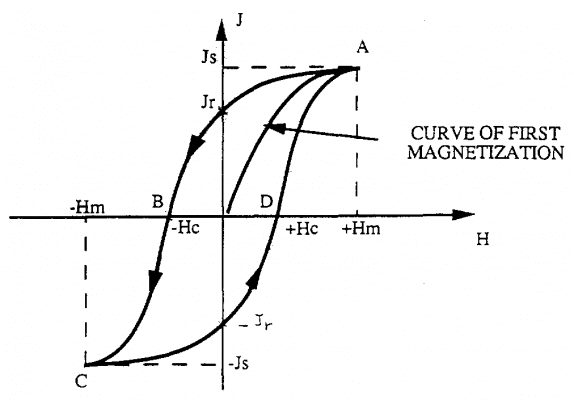 The closed curve ABCD, obtained with the change of the magnetic field H between the values of Hm and –Hm is called the hysteresis loop. The shape of the hysteresis ring depends on many factors, including the nature and composition of the ferromagnetic material and the mechanical processing; heat treatment of materials. Therefore, there are ferromagnetic types with large residual magnetization, while other types have small residual magnetization.
The closed curve ABCD, obtained with the change of the magnetic field H between the values of Hm and –Hm is called the hysteresis loop. The shape of the hysteresis ring depends on many factors, including the nature and composition of the ferromagnetic material and the mechanical processing; heat treatment of materials. Therefore, there are ferromagnetic types with large residual magnetization, while other types have small residual magnetization.
 II.6. Curie temperature
Any ferromagnetic material containing residual magnetism can be demagnetized by heating it to a suitable temperature and then cooling it in the absence of a magnetic field. The temperature at which a material changes its properties from a magnetic state to a non-magnetic (paramagnetic) state is called the temperature or Curie point. That temperature depends on the properties and composition of the material. For example, a Nickel alloy containing 1 % Silicon has a Curie temperature of 3200C. Nickel alloy containing 5% Silicon will have a Curie temperature of 450C. Curie temperature of steel varies from 6500C to 8700C. This phenomenon is reversible. When the temperature of the material is higher than the Curie Temperature, the material becomes paramagnetic. When the temperature is lower than the Curie Temperature, the material becomes ferromagnetic again but loses all its residual magnetism.
II.6. Curie temperature
Any ferromagnetic material containing residual magnetism can be demagnetized by heating it to a suitable temperature and then cooling it in the absence of a magnetic field. The temperature at which a material changes its properties from a magnetic state to a non-magnetic (paramagnetic) state is called the temperature or Curie point. That temperature depends on the properties and composition of the material. For example, a Nickel alloy containing 1 % Silicon has a Curie temperature of 3200C. Nickel alloy containing 5% Silicon will have a Curie temperature of 450C. Curie temperature of steel varies from 6500C to 8700C. This phenomenon is reversible. When the temperature of the material is higher than the Curie Temperature, the material becomes paramagnetic. When the temperature is lower than the Curie Temperature, the material becomes ferromagnetic again but loses all its residual magnetism.